.
Algebraic expressions and equations normally come in integer or fraction form.
Examples:
1) 4x - 3 = x
2) (3/4)x - 3x = 1/(3x)
Simplification of the above examples will not pose much of a problem except maybe in the challenge of bringing the numbers and unknowns over the "equal" sign.
But algebraic equations can come in decimal form too.
Example:
0.3(0.2x - 1) = 0.1x
How do we go about solving the above "decimated" algebraic equation easily?
A simple trick that I can think of (or maybe too simply a technique to call it 'trick").
What I would do is to multiply the expression on both sides by 10.
The idea is to bring the decimal number into the integer range.
BUT do note that the expression on the left side has two decimal numbers.
As such I would have to "x 10" twice.
This means that there is a "x 100" on the left and right side.
The new equation will thus be:
3 (2x - 10) = 10 x
==> 6x - 30 = 10x
==> -30 = 10x - 6x = 4x
==> x = -30 / 4 = -7.5
Conclusion:
Decimal can be seen to be intimidating when in the decimal form. However, it can be elevated to the familiar integer form through simple multiplication.
However, do take note of how many decimal number has been multiplied.
Left and right sides of the equation has to have the same number of multiplication (or division) to stay equal and valid.
Maths is not that frightening.
It can be interesting, if the method to "attack" it is properly done.
:-)
Wednesday, 8 December 2010
Monday, 22 November 2010
Basic Decimal Conversion
`
Every thing falls back to basic.
If the fundamentals are weak, any maths learners will have a hard time moving forward in their maths learning journey.
Let me quote an example.
How do we change 4.75 to fraction.
We can use 475 / 100 and reduce it through long division. This will give 4 and 3/4.
However, if we know that 4.75 is actually consisting of 4 add to 0.75, the conversion will be simpler.
4 and 0.75 means 4 + (3/4) which leads directly to 4 whole and 3/4. Same as answer of above.
(NOTE: 0.75 is a quarter which equates to 3/4).
Simple isn't it?
Maths is interesting.
:-)
Every thing falls back to basic.
If the fundamentals are weak, any maths learners will have a hard time moving forward in their maths learning journey.
Let me quote an example.
How do we change 4.75 to fraction.
We can use 475 / 100 and reduce it through long division. This will give 4 and 3/4.
However, if we know that 4.75 is actually consisting of 4 add to 0.75, the conversion will be simpler.
4 and 0.75 means 4 + (3/4) which leads directly to 4 whole and 3/4. Same as answer of above.
(NOTE: 0.75 is a quarter which equates to 3/4).
Simple isn't it?
Maths is interesting.
:-)
Labels:
applications,
Learning maths,
Number,
principles
Sunday, 3 October 2010
Pointers in Teaching, Learning Speed
.
At elementary level in maths education, speed is always a challenging topic for learners.
It caught my attention and I started wondering why?
Many mistakes can be made when dealing with these types of questions.
After studying the various mistakes made by learners, I came to a few conclusion that I like to share here.
How to avoid confusion in doing Speed questions in maths:-
1) Speed involves two parameters, namely, distance and time.
This is the key issue. Dealing with one parameter is already a challenge, and dealng with two is always a "headache".
The concept, has thus to be clearly addressed upon, before the ratio of distance and time leading to speed can be fully understood.
What is distance?
What is time?
These 2 items are variable in nature. They change in value.
They causes confusion when lumped together!
Examples of daily activities will help in this case.
Quote cases like running in a race, where the champion came back in the shortest time covering the same distance as all others.
Get the concept of distance versus time into them.
Also FAST and SLOW relation to speed.
2) Error in units:-
Break up the tasks of calculating km, m or cm and sec, hours, minutes separately.
In other words,deal with one item at a time.
Use basic unit if possible to reduce chances of making costly errors.
The learners have to handle the logical part of the question, and also the mechanical part of unit manipulation in speed problems.
Tell them to find one thing at a time, and the need for doing that. Be patience is the message.
3) Draw out a pictorial image of the question.
This method will help some kids to visualise the real issue.
By having drawn the length for distance to be covered (or covered), they will have a better idea of what distance is about in the maths question. They will not have to "keep" this disatnce in their mind together with the problematic "time" condition.
Use the seeing method helps them clear any doubts and can also reduce mistakes in interpreting the question.
There will definitely be more pointers to be added to my three above.
But with these 3 basic issues settled, most of the queries about speed and its maths problems should be clearer.
If you have any other pointers, you may share in the comment space.
Cheers :-)
Maths is interesting, I suppose you cannot agree more.
.
At elementary level in maths education, speed is always a challenging topic for learners.
It caught my attention and I started wondering why?
Many mistakes can be made when dealing with these types of questions.
After studying the various mistakes made by learners, I came to a few conclusion that I like to share here.
How to avoid confusion in doing Speed questions in maths:-
1) Speed involves two parameters, namely, distance and time.
This is the key issue. Dealing with one parameter is already a challenge, and dealng with two is always a "headache".
The concept, has thus to be clearly addressed upon, before the ratio of distance and time leading to speed can be fully understood.
What is distance?
What is time?
These 2 items are variable in nature. They change in value.
They causes confusion when lumped together!
Examples of daily activities will help in this case.
Quote cases like running in a race, where the champion came back in the shortest time covering the same distance as all others.
Get the concept of distance versus time into them.
Also FAST and SLOW relation to speed.
2) Error in units:-
Break up the tasks of calculating km, m or cm and sec, hours, minutes separately.
In other words,deal with one item at a time.
Use basic unit if possible to reduce chances of making costly errors.
The learners have to handle the logical part of the question, and also the mechanical part of unit manipulation in speed problems.
Tell them to find one thing at a time, and the need for doing that. Be patience is the message.
3) Draw out a pictorial image of the question.
This method will help some kids to visualise the real issue.
By having drawn the length for distance to be covered (or covered), they will have a better idea of what distance is about in the maths question. They will not have to "keep" this disatnce in their mind together with the problematic "time" condition.
Use the seeing method helps them clear any doubts and can also reduce mistakes in interpreting the question.
There will definitely be more pointers to be added to my three above.
But with these 3 basic issues settled, most of the queries about speed and its maths problems should be clearer.
If you have any other pointers, you may share in the comment space.
Cheers :-)
Maths is interesting, I suppose you cannot agree more.
.
Labels:
Learning maths,
speed
Sunday, 13 June 2010
Simple Logarithm Tip
.
Maths expression may at times look challenging, but a bit of a thought may make it otherwise.
Logarithm is always an exciting topics to new learners.
With the "log" coming into the maths expression, one will be confused.
Definitely!
But do rest assure, as the tip below shows.
Maths Tip
eln y = y
Proving this:
"Natural log" both sides will give ln eln y = ln y
Applying the law that ln an = n ln a, and that ln e = 1, you will notice that the above mathematical expressions are true and equal.
NOTE:
This tip applies to "log" too.
10log y = y
Hope this helps.
:-)
Maths expression may at times look challenging, but a bit of a thought may make it otherwise.
Logarithm is always an exciting topics to new learners.
With the "log" coming into the maths expression, one will be confused.
Definitely!
But do rest assure, as the tip below shows.
Maths Tip
eln y = y
Proving this:
"Natural log" both sides will give ln eln y = ln y
Applying the law that ln an = n ln a, and that ln e = 1, you will notice that the above mathematical expressions are true and equal.
NOTE:
This tip applies to "log" too.
10log y = y
Hope this helps.
:-)
Labels:
Logarithm
Wednesday, 2 June 2010
Model versus Variable Technique
'
In using Model method of solving maths question, we are using visual blocks to scope our thinking. This is followed by analysis through the models.
Models become a link to our thinking process.
The demerit is when we did not create the Model properly, or miss out some details that cause the model to be represented wrongly.
The merit is that it can be simple and straight forward when drawn properly. It reflects outright the relationship between many unknowns.
Less workings is thus needed, as visual que sets in.
For algebraic variable technique, the unknowns are pre-defined and booked as "letters". A space, mentally, has been reserved for the answer.
The working is just simply to accept that the answer is already there but only not numerical. Following through the working steps will ultimately reveal the letter of its numerical data which is what we want.
Variable as letter is good in the sense that we need less analysis, but just mechanically following the rules and steps leading to the final step, of course with some logic and mathematical strategy.
Each has its own advantages and weakness. It is up to us to make use of them in the correct way.
Experience is the only way to overcome the proper selection of which technique.
Thus practice to gain experience in maths is one good way to master maths.
Skiving is a no-no.
Through practice, you will sooner or later find that maths is interesting.
:-)
In using Model method of solving maths question, we are using visual blocks to scope our thinking. This is followed by analysis through the models.
Models become a link to our thinking process.
The demerit is when we did not create the Model properly, or miss out some details that cause the model to be represented wrongly.
The merit is that it can be simple and straight forward when drawn properly. It reflects outright the relationship between many unknowns.
Less workings is thus needed, as visual que sets in.
For algebraic variable technique, the unknowns are pre-defined and booked as "letters". A space, mentally, has been reserved for the answer.
The working is just simply to accept that the answer is already there but only not numerical. Following through the working steps will ultimately reveal the letter of its numerical data which is what we want.
Variable as letter is good in the sense that we need less analysis, but just mechanically following the rules and steps leading to the final step, of course with some logic and mathematical strategy.
Each has its own advantages and weakness. It is up to us to make use of them in the correct way.
Experience is the only way to overcome the proper selection of which technique.
Thus practice to gain experience in maths is one good way to master maths.
Skiving is a no-no.
Through practice, you will sooner or later find that maths is interesting.
:-)
Labels:
Algebra,
Learning maths,
maths technique
Thursday, 27 May 2010
Purpose of Variables in Algebra
'
Unknowns are literally unknowns.
In maths, these unknowns are a cuause formaths anxiety.
When you are in unfamiliar territory, you will naturally be uncomfortable and unease.
This is the same feelingwhen dealing with unknowns in maths.
Algebra came to the rescue for this problems.
Here, you will find unknowns named as "variables".
They served as "parking lots" for the final answers or unknowns.
In this algebra, you replace the variables for final numbers and work with them as though you already know them.
You simply go through the motion of solving the question with any given condition and numbers / data.
Upon finally reaching the last step, the numerical answers for the problem will be revealed.
This is the power of the variables in algebra.
Just simply work along, and not be fearful of the unknowns.
The steps will align you to the final answers.
Cheers!
Maths is interesting!
.
Unknowns are literally unknowns.
In maths, these unknowns are a cuause formaths anxiety.
When you are in unfamiliar territory, you will naturally be uncomfortable and unease.
This is the same feelingwhen dealing with unknowns in maths.
Algebra came to the rescue for this problems.
Here, you will find unknowns named as "variables".
They served as "parking lots" for the final answers or unknowns.
In this algebra, you replace the variables for final numbers and work with them as though you already know them.
You simply go through the motion of solving the question with any given condition and numbers / data.
Upon finally reaching the last step, the numerical answers for the problem will be revealed.
This is the power of the variables in algebra.
Just simply work along, and not be fearful of the unknowns.
The steps will align you to the final answers.
Cheers!
Maths is interesting!
.
Labels:
Algebra,
maths anxiety,
maths symbols
Saturday, 24 April 2010
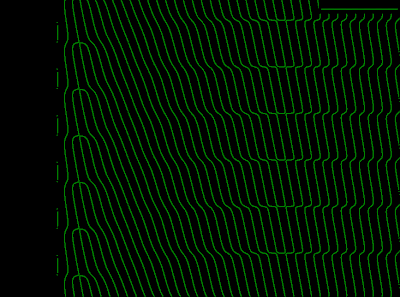
A Mathematical Waterfall
'
Mathematics equation can be fun.
It is not only used as a problem-solving tool, it can be used to create visual image simulating scene.
By trying a few equations, anyone with patience and basic maths knowledge can do it.
Simply create an expression or equation in a graph and tweet it to form any image.
Here you will see an image formed up to look like a waterfall.
Enjoy yourself.
This was done with both logarithm and trigonometry functions.
:-)
Mathematics equation can be fun.
It is not only used as a problem-solving tool, it can be used to create visual image simulating scene.
By trying a few equations, anyone with patience and basic maths knowledge can do it.
Simply create an expression or equation in a graph and tweet it to form any image.
Here you will see an image formed up to look like a waterfall.
Enjoy yourself.
This was done with both logarithm and trigonometry functions.
:-)
Labels:
graph,
graphical art,
Trigonometry
Wednesday, 14 April 2010
Maths Symbol in Our Applications
.
There are many symbols in maths.
To learn and understand maths, we need to know the meaning of the symbols.
This is very much like talking to a foreigner. Without understanding each other's language, no communication can be carried out (other than the international body language!)
Hence, knowing the usage of the symbols in a mathematical expression helps.
But is it really so?
Partially.
Why do I say that?
Yes, you may know the symbol while doing maths, but if the same symbol is used elsewhere, do you still understand?
One example is:
y = x + 1
This means x is added by one and their total is represented by the variable "y".
This is for the maths operator "+".
But what about the expression x++ ?
This looks odd, isn't it?
To the maths learner, this may be a typo error, or something is missing.
"x++" is actually commonly used in C programming.
What it means is x = x + 1.
It is a short-cut way of writing the addition of x and replacing it by the same variable "x".
Thus this example showed the use of "+" in another application.
It is still maths in some sense, but written in another form.
Maths is therefore always around us. It is a matter of us applying them and understanding them.
Only by learning their "language", can we communicate with them.
Interesting? I bet you agree!
Other applications can be " += ", " :-) " and " x>>4 ".
Can you find their meaning?
:D
There are many symbols in maths.
To learn and understand maths, we need to know the meaning of the symbols.
This is very much like talking to a foreigner. Without understanding each other's language, no communication can be carried out (other than the international body language!)
Hence, knowing the usage of the symbols in a mathematical expression helps.
But is it really so?
Partially.
Why do I say that?
Yes, you may know the symbol while doing maths, but if the same symbol is used elsewhere, do you still understand?
One example is:
y = x + 1
This means x is added by one and their total is represented by the variable "y".
This is for the maths operator "+".
But what about the expression x++ ?
This looks odd, isn't it?
To the maths learner, this may be a typo error, or something is missing.
"x++" is actually commonly used in C programming.
What it means is x = x + 1.
It is a short-cut way of writing the addition of x and replacing it by the same variable "x".
Thus this example showed the use of "+" in another application.
It is still maths in some sense, but written in another form.
Maths is therefore always around us. It is a matter of us applying them and understanding them.
Only by learning their "language", can we communicate with them.
Interesting? I bet you agree!
Other applications can be " += ", " :-) " and " x>>4 ".
Can you find their meaning?
:D
Labels:
Algebra,
Learning,
maths applications,
Number
Saturday, 10 April 2010
Number of Answers | Common mistake
Maths can be tricky when you are not careful.
This is not to frighten you, though.
This post is just to remind you of the wonderful aspect of maths in covering all areas.
Below is an example of what I meant.
Let's take the quadratic eqaution solving as a starting point
x2 = 5x
x = 5x / x = 5 (Answer)
At first, this looks pretty fine. The answer, when substituted back, produces match of equation.
But this is actually not complete.
Those doing quadratic equation will know 2nd order (x2) equation evaluates to 2 answsers.
The answers may be the same though.
Now, if we approach it using another method, let's see the different.
x2 - 5x = 0
==> x (x - 5) = 0 , after factorising
==> x = 0 and (x - 5) = 0
==> x = 0 and x = 5
There are two answers now.
We had the x = 5 initially, but what about this new x = 0.
We have missed out on the x = 0 with the first mehtod. It looks OK then.
What happen?
It may be due to lack of experience handling this form of maths question.
The concept in solving quadratic equation is actually not limited to second order.
The hidden message is depending on the order, the number of answers will follow suit.
What I meant is :
2nd order gives 2 answers,
3rd order gives 3 answers,
4th order gives 4 answers, etc.
It is this verry message that maths learner should capture. Otherwise you will be tricked to give only one answer which leads you to "mistakes" of being incomplete.
I agree that this is tricky, but within reasonable argument.
If a student practice hard (and smart), he will not fall prey to this type of simple math problem.
Do not get con again.
Enjoy maths. It's fun and interesting.
:D
This is not to frighten you, though.
This post is just to remind you of the wonderful aspect of maths in covering all areas.
Below is an example of what I meant.
Let's take the quadratic eqaution solving as a starting point
x2 = 5x
x = 5x / x = 5 (Answer)
At first, this looks pretty fine. The answer, when substituted back, produces match of equation.
But this is actually not complete.
Those doing quadratic equation will know 2nd order (x
The answers may be the same though.
Now, if we approach it using another method, let's see the different.
x2 - 5x = 0
==> x (x - 5) = 0 , after factorising
==> x = 0 and (x - 5) = 0
==> x = 0 and x = 5
There are two answers now.
We had the x = 5 initially, but what about this new x = 0.
We have missed out on the x = 0 with the first mehtod. It looks OK then.
What happen?
It may be due to lack of experience handling this form of maths question.
The concept in solving quadratic equation is actually not limited to second order.
The hidden message is depending on the order, the number of answers will follow suit.
What I meant is :
2nd order gives 2 answers,
3rd order gives 3 answers,
4th order gives 4 answers, etc.
It is this verry message that maths learner should capture. Otherwise you will be tricked to give only one answer which leads you to "mistakes" of being incomplete.
I agree that this is tricky, but within reasonable argument.
If a student practice hard (and smart), he will not fall prey to this type of simple math problem.
Do not get con again.
Enjoy maths. It's fun and interesting.
:D
Labels:
Algebra,
concept,
maths technique,
mistakes,
principles
Monday, 5 April 2010
Simultaneous Equations | Re-write equations
*
Simple simultaneous equation problem comes as 2 straight forward mathematical expressions.
Example 1:
3x + y = 4
x + 2y = 3
But some may come in odd expressions (since life is always the case, which makes learning maths more exciting!)
Example 2:
(12 - x)(1 + y) = 15
(8 - x) (1 + y) = -15
Here you will notice that the unknowns are biased towards one side.
Approach 1:
Multiply the 2 factors to get something like example 1.
Using elimination method, remove one of the unknown.
Solve for the only one unknown left.
Using the result found, compute the other unknown.
Approach 2: (The focus of this post)
Re-write the expression to make it look simpler.
The example 2 can be re-written into below simpler form:
(12 - x) = 15 / (1 + y) ===> (A)
(8 - x) = -15 / (1 + y) ===> (B)
Equation (B) can then be seen to be the negative of equation (A).
With the re-writing, we will be visually aided to see another form, a simpler one, of the simultaneous equations.
Moving forward with the solution...
12 - x = -(8 - x) = -8 + x
12 + 8 = 2x
x = 20 / 2 = 10 (ANSWER)
Putting x = 10 back into either equation (A) or (B),
We will get (12 - 10) (1 + y) = 15, if we select equation (A)
1 + y = 15 / 2 = 7.5
y = 7.5 - 1 = 6.5 (ANSWER)
The solution is not the issue in this post.
The key message here is the technique of "re-writing" the equations to reveal the simplicity of the question.
Maths is not that difficult if you look and think to make it easy.
Cheers!
.
Simple simultaneous equation problem comes as 2 straight forward mathematical expressions.
Example 1:
3x + y = 4
x + 2y = 3
But some may come in odd expressions (since life is always the case, which makes learning maths more exciting!)
Example 2:
(12 - x)(1 + y) = 15
(8 - x) (1 + y) = -15
Here you will notice that the unknowns are biased towards one side.
Approach 1:
Multiply the 2 factors to get something like example 1.
Using elimination method, remove one of the unknown.
Solve for the only one unknown left.
Using the result found, compute the other unknown.
Approach 2: (The focus of this post)
Re-write the expression to make it look simpler.
The example 2 can be re-written into below simpler form:
(12 - x) = 15 / (1 + y) ===> (A)
(8 - x) = -15 / (1 + y) ===> (B)
Equation (B) can then be seen to be the negative of equation (A).
With the re-writing, we will be visually aided to see another form, a simpler one, of the simultaneous equations.
Moving forward with the solution...
12 - x = -(8 - x) = -8 + x
12 + 8 = 2x
x = 20 / 2 = 10 (ANSWER)
Putting x = 10 back into either equation (A) or (B),
We will get (12 - 10) (1 + y) = 15, if we select equation (A)
1 + y = 15 / 2 = 7.5
y = 7.5 - 1 = 6.5 (ANSWER)
The solution is not the issue in this post.
The key message here is the technique of "re-writing" the equations to reveal the simplicity of the question.
Maths is not that difficult if you look and think to make it easy.
Cheers!
.
Labels:
concept,
maths technique,
simultaneous equations
Friday, 2 April 2010
Tips on Avoiding Mistakes (Unit writing)
.
Maths involves many traps.
Any one of this traps will make the solution looks odd or even to the extent of wrong answer.
What are this traps ?
Mathematical operators, symbols, units, transferring of numbers, size of the written symbols, decimal points are some of the examples of traps contributing to the error.
Here I would like to mention about "unit".
In maths, calculation of items are aplenty. One of them is the study of speed.
In the topic of speed, students are dealing with three basic elements.
They are the distance, time and their ratio (speed).
All these three elements have different units all to themselve.
Distance == metre
Time == second
Speed == metre / sec
There are variations of the above.
km, mintues, hours, km / h, m / min, etc
Do you now see the danger?
If you are dealing with so many units in one maths question, what are the chance of making mistakes?
If you are careful, the chance is low, but it does not mean zero.
You still have to be careful.
How to avoid having mistakes due to this undesired slip?
One tip is to write down the units in the working steps.
Do not leave the numerical answer (in the working) without any unit indicated.
Make clear the item of interest, whether it is distance or time by reflecting the unit besides the number.
Example: 5 km, 40 sec.
A complete maths example will push the message across, thus ....
Example :
Alan travelled at a speed of 60 km / h for 2 h. After that, he slowed down by 20 km / h and travelled the last quarter of the journey at this new speed. How long did he take to travel?
Working:
60 x20 = 120
120 / 3 = 40
60 - 20 = 40
40 / 40 = 1
2 + 1 = 3
Answer: 3 hrs.
What is your comment on the working?
I personally feel uncomfortable. What about you?
The danger in that sort of working is the lack of showing the actual item in the calculation.
It does not allow a good way for checking after completing the worksheet (if many maths problems are within).
Clearly writing the units will, at least, make checking later an easier task.
It also allows the marker (teacher) a clearer picture instead of guessing what you intend to show.
Along the way, during the working, you will also have a lesser chance of getting confuse as the items are listed with the proper message (through the units).
So are you convince proper unit presentation is worth the while?
A pointer for your thoughts.....
Cheers :-D
.
Maths involves many traps.
Any one of this traps will make the solution looks odd or even to the extent of wrong answer.
What are this traps ?
Mathematical operators, symbols, units, transferring of numbers, size of the written symbols, decimal points are some of the examples of traps contributing to the error.
Here I would like to mention about "unit".
In maths, calculation of items are aplenty. One of them is the study of speed.
In the topic of speed, students are dealing with three basic elements.
They are the distance, time and their ratio (speed).
All these three elements have different units all to themselve.
Distance == metre
Time == second
Speed == metre / sec
There are variations of the above.
km, mintues, hours, km / h, m / min, etc
Do you now see the danger?
If you are dealing with so many units in one maths question, what are the chance of making mistakes?
If you are careful, the chance is low, but it does not mean zero.
You still have to be careful.
How to avoid having mistakes due to this undesired slip?
One tip is to write down the units in the working steps.
Do not leave the numerical answer (in the working) without any unit indicated.
Make clear the item of interest, whether it is distance or time by reflecting the unit besides the number.
Example: 5 km, 40 sec.
A complete maths example will push the message across, thus ....
Example :
Alan travelled at a speed of 60 km / h for 2 h. After that, he slowed down by 20 km / h and travelled the last quarter of the journey at this new speed. How long did he take to travel?
Working:
60 x20 = 120
120 / 3 = 40
60 - 20 = 40
40 / 40 = 1
2 + 1 = 3
Answer: 3 hrs.
What is your comment on the working?
I personally feel uncomfortable. What about you?
The danger in that sort of working is the lack of showing the actual item in the calculation.
It does not allow a good way for checking after completing the worksheet (if many maths problems are within).
Clearly writing the units will, at least, make checking later an easier task.
It also allows the marker (teacher) a clearer picture instead of guessing what you intend to show.
Along the way, during the working, you will also have a lesser chance of getting confuse as the items are listed with the proper message (through the units).
So are you convince proper unit presentation is worth the while?
A pointer for your thoughts.....
Cheers :-D
.
Tuesday, 30 March 2010
Using Equation To Create A Square Graphically
'
While studying maths, I have been exposed to equation that forms a circle.
We know that x^2 + y^2 = 1 creates a circle.
But I have been wondering what is an equation to form a square.
I had tried a few mathematical expressions till today.
And finally I found the interesting and mysteries equation.
It utilises the same concept as the circle except that hyperbolic trigonometry is applied.
Below is a graph plotted with that equation.
The corners are rounded though. Any one has any try with a more sharper corner?
Graph is a wonderful tool as it can present results visually with one view.
Appreciating maths and using it appropriately can reduce many complex problems.
Maths is interesting.
.
While studying maths, I have been exposed to equation that forms a circle.
We know that x^2 + y^2 = 1 creates a circle.
But I have been wondering what is an equation to form a square.
I had tried a few mathematical expressions till today.
And finally I found the interesting and mysteries equation.
It utilises the same concept as the circle except that hyperbolic trigonometry is applied.
Below is a graph plotted with that equation.
The corners are rounded though. Any one has any try with a more sharper corner?
Graph is a wonderful tool as it can present results visually with one view.
Appreciating maths and using it appropriately can reduce many complex problems.
Maths is interesting.
.
Labels:
applications,
concept,
graph,
graphical art,
maths technique,
Trigonometry
Saturday, 27 March 2010
Area Displacement Theory
.
Maths is not all about calculation.
There are always more to it than meet the eyes.
This is especially true when you are doing geometrical questions where you are involved with area, perimeter and so on.
Displacement theory or its equivalent is always done without the knowledge of many people.
What is this theory about?
Let's look at one example below.
In the diagram above, you will see a path (white coloured) going across a blue platform.
If you are asked to find the area of this path, what can you do to obtain this area?
If no data of dimension is given, it is definitely not possible.
Now if the width of the path and the vertical length of the blue platform is given, can you compute the answer?
Again , this need a bit of thinking.
Displacement theory kicks in here. Look at the diagram on the right.
It is the displaced or closed up portion of the blue platform that does the trick.
Here you will notice the dashed line forming a white rectangluar area on the right-most side of the white blue platform.
Are you able to find the area of this white rectangular piece?
The width of this rectangle piece is ACTUAL the width of the white path!
You should now be able to calculate the area of this rectangular piece since the path width and length of the rectangular block is known or deduced now.
How this is possibe is through the "hidden" clue or step of closing up the path revealing the simpler rectangular area that any decent maths student can calculate.
Hence, maths is wonderful in that it tests you not only about applcations of maths tools, but your other "intelligence".
Having known displacement theory here, I believe you are really for the Math Challenge 23.
Go there and answer the question, and be quick before others grap the position one ...
:-D
.
Maths is not all about calculation.
There are always more to it than meet the eyes.
This is especially true when you are doing geometrical questions where you are involved with area, perimeter and so on.
Displacement theory or its equivalent is always done without the knowledge of many people.
What is this theory about?
Let's look at one example below.
In the diagram above, you will see a path (white coloured) going across a blue platform.
If you are asked to find the area of this path, what can you do to obtain this area?
If no data of dimension is given, it is definitely not possible.
Now if the width of the path and the vertical length of the blue platform is given, can you compute the answer?
Again , this need a bit of thinking.
Displacement theory kicks in here. Look at the diagram on the right.
It is the displaced or closed up portion of the blue platform that does the trick.
Here you will notice the dashed line forming a white rectangluar area on the right-most side of the white blue platform.
Are you able to find the area of this white rectangular piece?
The width of this rectangle piece is ACTUAL the width of the white path!
You should now be able to calculate the area of this rectangular piece since the path width and length of the rectangular block is known or deduced now.
How this is possibe is through the "hidden" clue or step of closing up the path revealing the simpler rectangular area that any decent maths student can calculate.
Hence, maths is wonderful in that it tests you not only about applcations of maths tools, but your other "intelligence".
Having known displacement theory here, I believe you are really for the Math Challenge 23.
Go there and answer the question, and be quick before others grap the position one ...
:-D
.
Labels:
area,
concept,
Geometry,
Learning maths,
maths applications,
principles
Wednesday, 24 March 2010
Percentage Increase in Area
.
Is there any formula or maths expression showing the ncrease in area when its length and its breadth are increase by m% ?
If you cannot find one, it does not matter. You can easily derive one!
Let us work on this and show the others how simple maths can help us solve daily issue.
Let the length be x and breadth be y.
If x and y increase by m%,
length becomes x + x(m/100), and breadth becomes y + y(m/100).
Area is length x breadth.
Thus new area becomes [ x + x(m/100)] [ y + y(m/100)]
This gives us an area of xy + (m/100)xy + (m/100)xy + (m/100)(m/100)xy.
From the above maths expression, we can deduce that increase in area is:
2 x m% + (m% x m%)/100
Example with numbers will convince readers better, therefore ......
Example
If the increase in perimeter is 10%, what is the increase in area?
Answer is 2 (10%) + (10% x 10%) /100 = 20% + 1% = 21%
Easy isn't it?
For other post related to this concept in percentage increase, see the post Percentage Increase in Perimeter.
Maths is interesting.
;-D
.
Is there any formula or maths expression showing the ncrease in area when its length and its breadth are increase by m% ?
If you cannot find one, it does not matter. You can easily derive one!
Let us work on this and show the others how simple maths can help us solve daily issue.
Let the length be x and breadth be y.
If x and y increase by m%,
length becomes x + x(m/100), and breadth becomes y + y(m/100).
Area is length x breadth.
Thus new area becomes [ x + x(m/100)] [ y + y(m/100)]
This gives us an area of xy + (m/100)xy + (m/100)xy + (m/100)(m/100)xy.
From the above maths expression, we can deduce that increase in area is:
2 x m% + (m% x m%)/100
Example with numbers will convince readers better, therefore ......
Example
If the increase in perimeter is 10%, what is the increase in area?
Answer is 2 (10%) + (10% x 10%) /100 = 20% + 1% = 21%
Easy isn't it?
For other post related to this concept in percentage increase, see the post Percentage Increase in Perimeter.
Maths is interesting.
;-D
.
Tuesday, 23 March 2010
Percentage Increase in Perimeter
.
Percentage is a nice and mystery word in maths.
Why do I say that?
Look at the example below:
If the perimeter has increased by 30%, does the length also increases by the same amount?
The answer is obviously YES.
Next,
If the perimeter is increased by 30%, does the area covered by it also increases by the same amount?
??? The answer needs some pondering, right?
Answer to this:
If the perimeter is increased by 30%, the length and width will both increase by 30%.
This makes the area increase by 2(30%) + (30% x 30%) = ?
(I will explain this maths calculation in a later post.)
For now, let's concentrate on the maths operation.
What do you get from 30% x 30%?
30% = 0.3
Thus 30% x 30% = 0.3 x 0.3 = 0.09 = 9%
This is a potential mathematical mistake.
Error: 30% x 30% = 900% !
So, increase in area becomes 60% + 9% = 69%
Interesting how the mind works.
If the mind is not clear when doing maths, common mistakes do occur.
With more practice, however, this form of mistakes will be lesser.
Hence, be careful when dealing with parameter such as perimeter, length and AREA.
Know their relation and be aware of the "catch" when this type of maths question is being asked.
Do not fall for the maths trick.
:-)
.
Percentage is a nice and mystery word in maths.
Why do I say that?
Look at the example below:
If the perimeter has increased by 30%, does the length also increases by the same amount?
The answer is obviously YES.
Next,
If the perimeter is increased by 30%, does the area covered by it also increases by the same amount?
??? The answer needs some pondering, right?
Answer to this:
If the perimeter is increased by 30%, the length and width will both increase by 30%.
This makes the area increase by 2(30%) + (30% x 30%) = ?
(I will explain this maths calculation in a later post.)
For now, let's concentrate on the maths operation.
What do you get from 30% x 30%?
30% = 0.3
Thus 30% x 30% = 0.3 x 0.3 = 0.09 = 9%
This is a potential mathematical mistake.
Error: 30% x 30% = 900% !
So, increase in area becomes 60% + 9% = 69%
Interesting how the mind works.
If the mind is not clear when doing maths, common mistakes do occur.
With more practice, however, this form of mistakes will be lesser.
Hence, be careful when dealing with parameter such as perimeter, length and AREA.
Know their relation and be aware of the "catch" when this type of maths question is being asked.
Do not fall for the maths trick.
:-)
.
Wednesday, 17 March 2010
Hidden Clues in Maths Questions
There are different levels in any educational system.
This goes with the learning of mathematics too.
At various level of learning, you will be presented with different level of complexity.
At the elementary stage, you will be shown maths questions that are real straight forward type.
At intermediate, a bit of mind twisting has to be done to resolve any challenge.
At the highest level, the questions come embedded with hidden clues to be discovered by learners and used to continue with the solving process.
But hidden clues are now becoming the norm among intermediate level due to its benefits to prevent pure memorising of mathematical technique.
A example of this interesting "hidden clue" can be seen in my Math Challenge 23.
There anyone taking up the challenge needs another step in order to "see" through the simple trick of solving the issue.
(Note: The challenge requires only one step to calculate the area of the path).
Multi-discipline is thus needed for merit of helping get the answer.
Knowledge in utilising maths tools and technique are not sufficient these days.
Maths students have to know some basic theory of motional replacement to understand Math Challenge 23.
Hence, to master mathematics, it will be good to read more, especially, topics outside maths.
This enlarge your understanding of real-life cases roped into maths questions.
Maths is interesting in this manner since it involves not only one learning discipline but encompasses more.
Enjoy maths. It widens your perspective of the world.
:-)
This goes with the learning of mathematics too.
At various level of learning, you will be presented with different level of complexity.
At the elementary stage, you will be shown maths questions that are real straight forward type.
At intermediate, a bit of mind twisting has to be done to resolve any challenge.
At the highest level, the questions come embedded with hidden clues to be discovered by learners and used to continue with the solving process.
But hidden clues are now becoming the norm among intermediate level due to its benefits to prevent pure memorising of mathematical technique.
A example of this interesting "hidden clue" can be seen in my Math Challenge 23.
There anyone taking up the challenge needs another step in order to "see" through the simple trick of solving the issue.
(Note: The challenge requires only one step to calculate the area of the path).
Multi-discipline is thus needed for merit of helping get the answer.
Knowledge in utilising maths tools and technique are not sufficient these days.
Maths students have to know some basic theory of motional replacement to understand Math Challenge 23.
Hence, to master mathematics, it will be good to read more, especially, topics outside maths.
This enlarge your understanding of real-life cases roped into maths questions.
Maths is interesting in this manner since it involves not only one learning discipline but encompasses more.
Enjoy maths. It widens your perspective of the world.
:-)
Labels:
applications,
concept,
Geometry,
maths anxiety,
maths technique
Sunday, 14 March 2010
Math Challenge 23
*
Albert needed to create a path through a garden of his.
The garden has a size of a rectangle with length of 20m and width of 15m.
He intend to have a path of 3m wide.
His design is shown below.
But he has a problem.
He wanted to know what is the area of this path he is going to lay across the garden.
Can anyone help him calculate that area?
Basic geometry knowledge may helps.
Albert needed to create a path through a garden of his.
The garden has a size of a rectangle with length of 20m and width of 15m.
He intend to have a path of 3m wide.
His design is shown below.
But he has a problem.
He wanted to know what is the area of this path he is going to lay across the garden.
Can anyone help him calculate that area?
Basic geometry knowledge may helps.
Labels:
Geometry,
Maths Thinker
Wednesday, 24 February 2010
Math Challenge 22
*
Given the diagram of boxes below, determine, in the fastest possible way, the area of the dark blue region.
Assume the individual boxes to be 1 unit square in area.
Given the diagram of boxes below, determine, in the fastest possible way, the area of the dark blue region.
Assume the individual boxes to be 1 unit square in area.
Give your answer in the comment space, please.
Maths does not involve plain counting.
It involves some form of intelligence to get things going.
:-)
.
Labels:
Geometry,
Maths Thinker
Wednesday, 3 February 2010
Artistic Mathematical Lantern
'
Have you wondered what can maths do to art?
Below is a lantern made by a mathematical expression.
With maths, you can be assured of producing wonderful images when you have the appropriate expression.
Do create some for enjoyment.
Maths is interesting.
:-)
Have you wondered what can maths do to art?
Below is a lantern made by a mathematical expression.
With maths, you can be assured of producing wonderful images when you have the appropriate expression.
Do create some for enjoyment.
Maths is interesting.
:-)
Labels:
Fun in maths,
graphical art
Friday, 29 January 2010
Maths Solution Presentation
'
To get good marks for a maths test requires understanding of how teacher marks the paper.
"Why do I not get full marks when I have the correct numerical answers?".
This is a common question at the back of any maths students when they see marks deducted "illogically".
Explanation:
When maths teacher give a maths question, she will like to know how is the answer obtained.
She wants to know whether the "thinking" part of solving the problem existed.
With the objectives in mind, the marking schemes are sometimes created to have marks for every steps involved in getting the answer.
Thus getting the answer without the required steps, even though it is mental, is a no-no.
Let me give an example.
Solve (x + 1)(x - 4) = 0
Solution A:
x = -1
x = 4
Solution B:
x + 1 = 0 ===> x = 1
x - 4 = 0 ===> x = 4
Comparing the two solutions presented above, you will notice clearly that Solution B is a better presented solution with proper steps reflecting the "thinking" process of the students.
Though the student of Solution A has the answer correct, he did not reveal the steps and demonstrate his understanding.
With that lack of presentation, he lost precious marks.
However, do note that not every time, we need to write down every steps.
It depends on which educational level you are in.
For the above example of presentation, the level is that of elementary, where foundational understanding is a necessity.
Upon graduating to high school, less detailed steps are needed. This is because it is assumed that the students had obtained a certain level of mathematical computing skill to that level of studies.
As such, reflection of the internal thinking to show minor details can be ignored and "by-passed" to shorten solution time.
However, the marks will still be given for steps needed at high-school level.
This goes for university level too.
By then the marking scheme will access advance thinking steps rather the minor calculations.
When errors do occurs in the calculations, it will normally be taken as "human" error as opposed to conceptual error.
In summary, do know the necessary solution steps to present during test or important assignment. Do understand the requirement and objectives of the test.
Do know what is being tested.
Writing too little can be detrimental at a lower educational level.
And writing too much can be disastrous at higher level, since you will be left with little time to complete the paper.
Hence doing maths is not simply completing the paper and getting correct answers.
It is a total strategic plan involving a lot of soft skills besides the computational abilities.
Cheers to maths, and
Cheers to it being interesting!
.
To get good marks for a maths test requires understanding of how teacher marks the paper.
"Why do I not get full marks when I have the correct numerical answers?".
This is a common question at the back of any maths students when they see marks deducted "illogically".
Explanation:
When maths teacher give a maths question, she will like to know how is the answer obtained.
She wants to know whether the "thinking" part of solving the problem existed.
With the objectives in mind, the marking schemes are sometimes created to have marks for every steps involved in getting the answer.
Thus getting the answer without the required steps, even though it is mental, is a no-no.
Let me give an example.
Solve (x + 1)(x - 4) = 0
Solution A:
x = -1
x = 4
Solution B:
x + 1 = 0 ===> x = 1
x - 4 = 0 ===> x = 4
Comparing the two solutions presented above, you will notice clearly that Solution B is a better presented solution with proper steps reflecting the "thinking" process of the students.
Though the student of Solution A has the answer correct, he did not reveal the steps and demonstrate his understanding.
With that lack of presentation, he lost precious marks.
However, do note that not every time, we need to write down every steps.
It depends on which educational level you are in.
For the above example of presentation, the level is that of elementary, where foundational understanding is a necessity.
Upon graduating to high school, less detailed steps are needed. This is because it is assumed that the students had obtained a certain level of mathematical computing skill to that level of studies.
As such, reflection of the internal thinking to show minor details can be ignored and "by-passed" to shorten solution time.
However, the marks will still be given for steps needed at high-school level.
This goes for university level too.
By then the marking scheme will access advance thinking steps rather the minor calculations.
When errors do occurs in the calculations, it will normally be taken as "human" error as opposed to conceptual error.
In summary, do know the necessary solution steps to present during test or important assignment. Do understand the requirement and objectives of the test.
Do know what is being tested.
Writing too little can be detrimental at a lower educational level.
And writing too much can be disastrous at higher level, since you will be left with little time to complete the paper.
Hence doing maths is not simply completing the paper and getting correct answers.
It is a total strategic plan involving a lot of soft skills besides the computational abilities.
Cheers to maths, and
Cheers to it being interesting!
.
Labels:
attitude,
Learning maths
Friday, 8 January 2010
Proper Way Of Writing Maths Expression
*
Maths expression tells certain message. When it is not written properly, or written in such a way that it causes wrong interpretation, then you will expect marks to be deducted.
Examples:
1) y = cos (A + B)
2) g = x + log K
3) y / x + 2
Let's look at the above examples one by one.
Example 1:
If the brackets are taken out, y = cos A + B.
Does it also mean B + cos A?
Example 2:
If the sequence is swapped, y = log K + x
Does it mean y = log (K + x)?
Example 3:
Is the denominator just x or (x + 2)?
Or is the correct expression 2 + (y /x) ?
From the above 3 maths expressions, you will observe and sense that something will go wrong when you did not write "properly".
This need practice and does need some "maths" sense to go along with the practice.
You need to know the different form of expression and its implications.
Questions like:
- one term or two terms in the desired expression?
- which is the actual denominator?
- will anyone mis-interpret the logging of term?
- If the words or symbols are too small, will they be able to see clearly?
To save time and marks, write with the reader or marker at heart.
Write as though they are reading them.
Think and write like they will be.
Maths is afterall, a language that has to be shared and used to solve certain objectives.
Do write clearly and appropriately.
The practice and skill mastered will do you and everyone one good.
Strive to make less unnecessary mistakes and reduce the chance of your marks being subtracted off through improper writing.
Cheers! ^.^
.
Maths expression tells certain message. When it is not written properly, or written in such a way that it causes wrong interpretation, then you will expect marks to be deducted.
Examples:
1) y = cos (A + B)
2) g = x + log K
3) y / x + 2
Let's look at the above examples one by one.
Example 1:
If the brackets are taken out, y = cos A + B.
Does it also mean B + cos A?
Example 2:
If the sequence is swapped, y = log K + x
Does it mean y = log (K + x)?
Example 3:
Is the denominator just x or (x + 2)?
Or is the correct expression 2 + (y /x) ?
From the above 3 maths expressions, you will observe and sense that something will go wrong when you did not write "properly".
This need practice and does need some "maths" sense to go along with the practice.
You need to know the different form of expression and its implications.
Questions like:
- one term or two terms in the desired expression?
- which is the actual denominator?
- will anyone mis-interpret the logging of term?
- If the words or symbols are too small, will they be able to see clearly?
To save time and marks, write with the reader or marker at heart.
Write as though they are reading them.
Think and write like they will be.
Maths is afterall, a language that has to be shared and used to solve certain objectives.
Do write clearly and appropriately.
The practice and skill mastered will do you and everyone one good.
Strive to make less unnecessary mistakes and reduce the chance of your marks being subtracted off through improper writing.
Cheers! ^.^
.
Labels:
Learning maths,
mistakes
Subscribe to:
Posts (Atom)





+copy.jpg)






